Elastic Buckling Factor of Safety

The Elastic Buckling Factor of Safety is defined as:

where the Euler buckling stress is equal to:

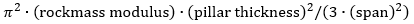
For this calculation, the roof is treated as a clamped beam, with span and breadth equal to the shorter and longer of the x and y dimensions, respectively.

The horizontal confining stress = the stress along the breadth of the beam (the longer dimension).

If the lateral stresses are very low, the elastic buckling Factor of Safety will be very high, and a Voussoir analysis may be more appropriate. Elastic buckling is only an issue when:

• Lateral stresses are high, and/or
• Span/depth ratio is high, and/or
• Rock mass modulus is low

To apply high lateral stresses to a model, you can either:

• Use the Constant stress option, which allows the actual horizontal effective stresses to be input, or
• Use the Gravity stress option, which allows the locked in stress to be input (lateral stress at the pillar surface).

Due to the inherent uncertainty in applying elastic buckling criteria to rock, a high mean Elastic Buckling Factor of Safety is recommended (e.g. at least > 3), if elastic buckling is an issue. In most situations however, the Elastic Buckling Factor of Safety will be high to very high.